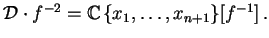Task:	Compute the roots of the (local) Bernstein polynomial  b = b(s), the minimal non-trivial complex polynomial, which satisfies
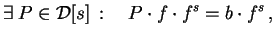	where
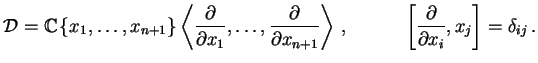	Here,   fs   is a formal notation for an indeterminate T on which we have the following actions:

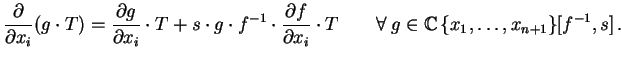Note that the roots of b are negative rational numbers and -1 is a root of b. To compute the roots of the Bernstein polynomial b excluding the root -1, we type:

ring R=0,(x,y),ds;
poly f=x5+x2y2+y5;
LIB "gaussman.lib";
bernstein(f); // an implementation based on Malgrange's results

==>

_[1]=-1/2 _[2]=-7/10 _[3]=-9/10 _[4]=-11/10 _[5]=-13/10

In particular, since -2 is smaller than all roots of the Bernstein polynomial of f, we have